Piledriving - Point, Line or Moving Source?

We've had a few questions on how to model pile driving. I think it's time to do a post on what sort of source geometry one can use for pile driving.

Piles are complex sources as they are not easily characterised as monopole sources (as e.g. an omnidirectional transducer would be). Even seismic arrays can, at sufficient distance, be treated fairly as a monopole source, given its relatively small size compared to the usual operating sites.

For piles this is rarely the case, they span the whole depth of the site (that's the point of piles) and emit sound along their whole length when struck or vibrated - more like a line source, or a very fast-moving source (speed equal to compressional wave speed of steel, >5000 m/s).

This is the case in theory, but I'm interested in the modelling application of this:

1. Can we safely model pile driving as a point source?
2. What other source geometries might be better suited?

I can already say that I'm not going to address "1" here as I'd need to compile quite a lot of recordings from the field and run models for each one (too time-consuming for a blog post).

I can, however, have a go at question "2":

Using four different bathymetry transects and three different source geometries we can get an idea of how they compare.

This post is only valid for comparing source geometries for SEL and/or SPL metrics.
Similar assessment for Lpk wil likely have a different outcome!

I ignore variations in sediment, soundspeed, temperature and other environmental variables.

Transects:

"5-12 m Rough"

This is from the North Sea in a shallow area with a gentle down slope - typical area for a wind farm - on top of a shallow area.

"41-77 m Rough"

Also from the North Sea, but quite a bit deeper. I only know of a few piles set this deep. The large drop in the middle is not a step, but rather a slope from 45-70 m depth over ~250 m, a 10 % gradient.

"17.5 m Flat"

This wouldn't be a modelling exercise without an impossibly smooth bottom.

"10-50 m Seamount"

Another classic in modelling, we introduce a large obstacle

• All sediment here were sand, (soundspeed 1650 m/s, density 1900 kg/m3, attenuation/wavelength 0.8, no shear waves).
• Isovelocity soundspeed profile.
•  dBSeaPE 16-1000 Hz, dBSeaRay 2-128 kHz
  

Source Geometries

1. Single point source
   This is a single point source placed at mid-depth.
   
   
2. Multiple point sources
   Imitating a line source by having multiple (17-48) point sources vertically arranged a the source location. Each point has had it's source level adjusted to reflect the number of source in the line.
   I.e. if there are 24 source point in the line each source's source level is equal to the single point source minus 10×log10(24) <=> single point - 13.8 dB.
   Or in general terms: SL-linepoint = SL-singlepoint - 10×log10(Npoints).
   
   
3. Moving source
   A moving source, approximated by a series of points (1 per meter) moving at 5000 m/s from a depth of 1 m to 1 m above the sediment.

Comparisons

I used fitted transmission loss function of the form TL=M×log10(range)+C, where M is the multiplication factor for the function that dictates the spreading loss, and M is a constant that offsets (up/down) to best fit the trend line. Transmission losses are the minimal transmission losses at any depth at the given horizontal range.

"5-12 m Rough".

"41-77 m Rough"

"17.5 m Flat"

"10-50 m Seamount" (log type curves are a bad fit on seamounts...)

Transmission losses for the 3 different source geometries and 4 different transect profiles are all very similar within their own scenario. Note that where there is most difference (ranges < 2000 m) the point source geometry has less or equal transmission loss (higher received levels).

We can see that the point source tends to generate a more erratic noise field, with larger fluctuation around the central tendency.

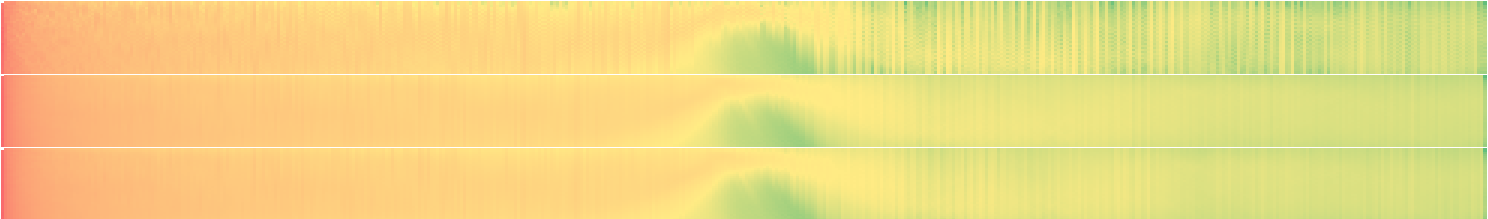Point (top), Moving (middle) and Line (bottom) source geometry for the seamount case. Note the more variable sound field with the point source geometry

From a practical modelling standpoint it makes sense to do a test like the above for your scenario to verify that the same pattern holds, as it will simplify and speed up your model.

I argue that the small gains in precision gained from a more complex source geometry are not significant when compared to the uncertainty of the other input parameters in a model, e.g. having a range dependent sound speed profile is likely to affect you results much more than source geometry.